Which is better, 7% or 8%?
Part of the investment process involves monitoring and evaluating your success, or better yet, the success of the counselor or advisor who is charged with the responsibility of handling your investments. Unfortunately, it can be quite common among professional athletes, TV personalities, and screen actors to lack the understanding of how to do this, the time to do this, or even the interest in doing this. And often times, worse, many athletes put unwavering trust in an advisor who may be smart enough to obscure the reality of what’s happening behind the scenes. As such, I ask again, would you rather average a 7% return or an 8% return? The correct answer is: that’s not enough information to draw a conclusion.
Now how could that be? Why would anyone prefer to accept a lower rate of return? The most obvious answer is that the investment having a higher average return may have necessitated the acceptance of greater risk. While that’s true, the risk factor still does not provide enough information to draw a conclusion to answer my question. In fact, in the interests of simplicity, let us assume that there is identical risk in these two hypothetical investments. I invite readers to re-assess why one may be happier with a 7% average return instead of an 8% average return.
And the reason is… Because averages can lie. That’s correct. Have you ever heard of the swimmer who drowned in a lake with an average depth of only two feet? Sure the lake may have an average depth of two feet, while the deepest part may be 30 feet. And herein lies the problem with investment professionals and mutual funds who advertise average annual returns.
I promise my readers not to bear down on mathematics and we really don’t need to. But I need to at least be sure that we understand what an “average” is. In our daily lives, we calculate averages all the time. If a player scores 10 points in one game and 30 points in another, we all know he/she is averaging 20 points. The actual equation is: The sum total of all numbers, divided by how many numbers there are. In this example, 10+30 = 40. Then we divide by 2 because we used two games. If a player scored in three games 10, 20, and 30 points, the average is obviously 20. The equation is: 10+20+30 = 60. Then we divide by 3 because we are discussing 3 games.
Now let’s get back to the point and talk about why averages in the investment world can be so terribly misleading.
Consider two $100 investments, each averaging a zero percent rate of return over two years. The first investment earns nothing in the first year, nothing in the second year, and thus concludes at the exact same value after two years: $100. The second investment earns 20% in the first year but unfortunately loses 20% in the second year. The problem here is that after year 1, this investment loses 20% of a larger number and therefore, the loss exceeds the previous year’s gain. Think about it—$100 up 20% equals $120; but then losing 20% of $120 causes a reduction in value of $24. So after year two, the second investment is worth $96 because it earned $20 but then lost $24.
And one can switch around the order of gain/loss and capture the same result. If the second investment looses 20% first, then gains 20%, the gain is 20% of a smaller number and therefore you do not recover your loss.
Both examples average a zero percent rate of return—one is even; one loses. If an investment advisor responsible for the second investment was asked what his two year results were, I fear that some might say “We averaged 0%,” but in reality “the client lost money.”
A scary real-world example I’ve shown clients is Intel Common Stock from the end of 2001 through the end of 2004. As we see in the table below, over that time period, Intel averaged +9.44%. However, if you had invested one million dollars, you would have lost over a quarter of a million bucks during that period! How is that possible? How could an investor average +9.44% but actually lose 25% of their investment?? See below…
*Chart based on the price of Intel Corp. common stock from 12/31/2001 through 12/32/2004. Yr. Return is the percentage price change between dates shown.
Let's use this logic and return to my original question. Would you rather average a 7% return or an 8% return? In order to effectively draw a conclusion, we need to study the expected volatility. If the ups and downs are significant, any gains may be insufficient to compensate for the losses experienced. Although average returns are only one way to calculate the progress of an investment, it really makes me wonder why in some cases, it is a regulatory requirement to show averages when they can be so horrifically misleading.
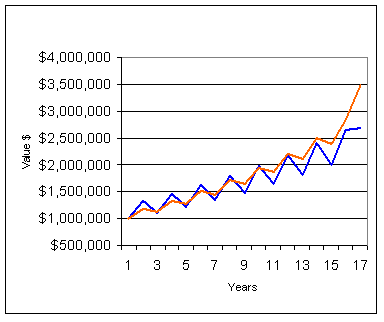
In the two graphs shown below, we see two investments—one with an annual average rate of return of 8%, the other 7%. As you can see, due to the extreme volatility of the portfolio averaging 8%, the gains are not enough to offset the negative effect of the losses and the portfolio is incapable of keeping up with the less volatile portfolio with an average rate of return of 7% over time.
The charts above are hypothetical in nature and do not represent the performance of any particular investment. The blue numeric data and chart line represent a hypothetical non-specific portfolio with an average rate of return of 8%, The orange data and chart line represent a non-specific portfolio with an average rate of return of 7%. The charts do not take into account effect of fees or taxes. The charts are not intended to accurately represent an investment portfolio and are for educational purposes only.
The biggest issue here—and it clearly relates to professional athletes—is that people who subcontract their responsibilities may not take the time to learn and really understand what is happening behind the scenes. And dare I say that this issue can be worse among celebrities because their attention is drawn to many other places and the likelihood is that many of them have never had to learn this stuff because many have gone from rags to riches.
While finance and investments can be daunting, the most important thing to remember is: none of us can be experts in all things. With that in mind, be sure your counselor or advisor is trustworthy, and be certain that you are intimately involved in reviewing your financial plans, your tax returns, and your investment performance. You don’t have to be a wizard to be a participant. You only have to be present and interested in being present to be a participant. And if today’s balance is lower than yesteryears, ask why… and don’t stop asking until you are comfortable with the answer.
There is a famous quote that reads: “If you trust someone with $20, and never see that person again, it was probably worth it.” Unfortunately, most professional athletes are dealing with a bit more than 20 bucks.
The purpose of this article is to promote education because education can often increase safety. And as I always say: safety first, always.
Disclaimer:
Matt Ramer is a Financial Advisor, Vice President, and Senior Portfolio manager with the Global Wealth Management Division of Morgan Stanley Smith Barney in Philadelphia. The information contained in this article is not a solicitation to purchase or sell investments. Any information presented is general in nature and not intended to provide individually tailored investment advice. The strategies and/or investments referenced may not be suitable for all investors as the appropriateness of a particular investment or strategy will depend on an investor's individual circumstances and objectives. Investing involves risks and there is always the potential of losing money when you invest. The views expressed herein are those of the author and may not necessarily reflect the views of Morgan Stanley Smith Barney LLC, Member SIPC, or its affiliates.
.bmp)

.bmp)

